Surface Areas and Volumes Class 9 Case Study Questions Maths Chapter 11

Reading Time: 12 minutes

Last Updated on October 26, 2024 by XAM CONTENT

Hello students, we are providing case study questions for class 9 maths. Case study questions are the new question format that is introduced in CBSE board. The resources for case study questions are very less. So, to help students we have created chapterwise case study questions for class 9 maths. In this article, you will find case study questions for CBSE Class 9 Maths Chapter 11 Surface Areas and Volumes. It is a part of Case Study Questions for CBSE Class 9 Maths Series.

Chapter	Surface Areas and Volumes
Type of Questions	Case Study Questions
Nature of Questions	Competency Based Questions
Board	CBSE
Class	9
Subject	Maths
Useful for	Class 9 Studying Students
Answers provided	Yes
Difficulty level	Mentioned
Important Link	Class 9 Maths Chapterwise Case Study

Case Study Questions on Surface Areas and Volumes

Case Study Questions

Question 1:

For decoration purpose, Sneha bought 100 orbeez balls and put it in a cylindrical shaped box. After filling it with water, the orbeez ball swell up and completely filled the cylindrical shaped box. Behind the orbeez ball packet, the change in volume of each orbeez ball was mentioned and which was 32% increase. Suppose the volume of all orbeez ball is 9900 cm³.

On the basis of the above information, solve the following questions:

Q 1. Find the volume of each orbeez ball.

Q 2. What is the volume of orbeez ball before swelling?

Q 3. How many orbeez balls before swelling was needed to completely fill the cylindrical shaped box?

Q 4. What is the cubic radius of a orbeez ball before swelling up?

Q 5. If the change in volume of orbeez ball is increased to 48%, then find the volume of orbeez ball after swell up.

Solutions:

1. Volume of one orbeez ball $\times 100=$ Volume of all orbeez ball
$\therefore$ Volume of one orbeez ball $=\frac{9900}{100}=99 \mathrm{~cm}^3$

2. Let original volume of orbeez ball before swelling be $x$.
Now, change in volume of orbeez ball

$$
=\frac{99-x}{x} \times 100
$$

Given, $\quad 32=\frac{(99-x) \times 100}{x}$

$$
\begin{array}{rlrl}
\Rightarrow & 32 x & =9900-100 x \\
\Rightarrow & 132 x & =9900 \\
\Rightarrow & x & =\frac{9900}{132} \\
& \therefore & x & =75 \mathrm{~cm}^3
\end{array}
$$

3. $n \times$ Volume of one orbeez ball before swell up

$$
\begin{array}{lr}
\Rightarrow & n \times 75=9900 \\
\Rightarrow & n=\frac{9900}{75} \\
\therefore & n=132 \text { balls }
\end{array}
$$

$$
=\text { Volume of container }
$$

4. Volume of a orbeez ball before swell up $=75 \mathrm{~cm}^3$
$\Rightarrow \quad \frac{4}{3} \pi r^3=75$
$\Rightarrow \quad \frac{4}{3} \times \frac{22}{7} \times r^3=75$
$\Rightarrow \quad r^3=\frac{75 \times 3 \times 7}{4 \times 22}$
$\therefore \quad r^3=17.89 \mathrm{~cm}^3$

5. Let the volume of orbeez ball after swell up be $x$.

Then, $\quad 48=\frac{x-75}{75} \times 100$

$$
\begin{aligned}
& \Rightarrow \quad 48=\frac{x-75}{3} \times 4 \\
& \Rightarrow \quad 48 \times 3=4 \mathrm{x}-300 \\
& \Rightarrow \quad 4 x=144+300 \Rightarrow 4 x=444 \mathrm{~cm}^3 \\
& \therefore \quad x=111 \mathrm{~cm}^3
\end{aligned}
$$

Understanding Surface Areas and Volumes

Solid Figures: Three-dimensional figures having definite shape, size and occupying a fixed amount of space in three dimensions. e.g., cuboid, cylinder, cone, sphere etc.

Surface Area: The sum of areas of all the faces of a solid figure. Its unit is unit2 or square unit.

Volume: The space occupied by a solid. Its unit is unit3 or cubic unit.

Right Circular Cone: A solid generated by revolving a line segment that passes through a fixed point and makes a constant angle with a fixed
line. It has one curved face and one plane circular face. e.g., funnel, joker‘s cap, etc.

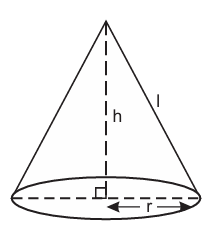
1. Volume $=\frac{1}{3} \pi r^2 h$

2. Curved Surface Area (CSA)

$$
\begin{aligned}
& =\frac{1}{2} \times l \times \text { Circumference of base } \\
& =\frac{1}{2} \times l \times 2 \pi r=\pi r l
\end{aligned}
$$

3. Total Surface Area (TSA) $=$ CSA + Area of the base

$$
=\pi r l+\pi r^2=\pi r(l+r)
$$

4. Slant height $(l)=\sqrt{h^2+r^2}$

Sphere: A solid figure made up of all points in space which lie at a constant distance called radius from a fixed point called the centre of the sphere. Let ‘r’ be the radius of sphere.

1. Volume $=\frac{4}{3} \pi r^3$

2. Curved Surface Area (CSA) $=$ Total Surface Area $(\mathrm{TSA})=4 \pi r^2$

Hemisphere: A plane through the centre of a solid sphere cuts it into two equal parts, each part is called a hemisphere. Let ‘r’ be the radius
of hemisphere.

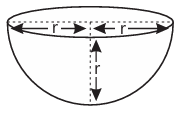
1. Volume $=\frac{2}{3} \pi r^3$

2. Curved Surface Area $(C S A)=2 \pi r^2$

3. Total Surface Area $($ TSA $)=$ CSA + Area of the base $=2 \pi r^2+\pi r^2=3 \pi r^2$

Hemispherical Shell: Let ‘R’ be the external radius and ‘r’ be the internal radius.

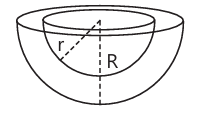
1. Area of base $=\pi\left(R^2-r^2\right)$

2. External CSA $=2 \pi R^2$

3. Internal CSA $=2 \pi r^2$

4. Volume of material $=\frac{2}{3} \pi\left(R^3-r^3\right)$

5. Thickness $=R-r$

Boost your knowledge

• The total surface area of any object is greater than its lateral surface area.
• When an object of certain volume is recast into a new shape, the volume of new shape formed will always be equal to the volume of the original object.
• The solids having the same curved surface do not necessarily occupy the same volume.
• When an object is dropped into a liquid, the volume of the displaced liquid is equal to the volume of the object that is dipped.
• The volume of the material in a hollow body is equal to the difference between the external volume and internal volume.
• To find the cost of polishing/covering/painting of a solid, we first find its surface area and then multiply by its per unit cost.
• To find the quantity of a substance contained in a solid, we find its volume.
• Volume of water accumulated on a roof after rain = Surface area of roof x Height of Rainfall.
• Volume of water released by a pipe in unit time = Rate of flow x Area of cross-section of pipe.

Also check

• Statistics Class 9 Case Study Questions Maths Chapter 12
• Surface Areas and Volumes Class 9 Case Study Questions Maths Chapter 11
• Heron’s Formula Class 9 Case Study Questions Maths Chapter 10
• Circles Class 9 Case Study Questions Maths Chapter 9
• Quadrilaterals Class 9 Case Study Questions Maths Chapter 8
• Triangles Class 9 Case Study Questions Maths Chapter 7
• Lines and Angles Class 9 Case Study Questions Maths Chapter 6
• Introduction to Euclid’s Geometry Class 9 Case Study Questions Maths Chapter 5
• Linear Equations in Two Variables Class 9 Case Study Questions Maths Chapter 4
• Coordinate Geometry Class 9 Case Study Questions Maths Chapter 3
• Polynomials Class 9 Case Study Questions Maths Chapter 2
• Number Systems Class 9 Case Study Questions Maths Chapter 1

Topics from which case study questions may be asked

• Surface Area of Sphere
• Surface Area of Hemisphere
• Volume of Sphere and Hemisphere
• Surface Area of Cone
• Volume of Cone
• Surface Area and Volume of Hemispherical Shell

To find the quantity of a substance contained in a solid, we find its volume.

Case study questions from the above given topic may be asked.

Helpful Links for CBSE Class 9 Preparation

• Download Chapter Tests for CBSE Class 9 Science
• Download Important MCQ Questions for CBSE Class 9 Physics
• Download Worksheets for CBSE Class 9 Science
• Download Case Study Questions for CBSE Class 9 Maths
• Download Sample Papers for CBSE Class 9
• Download HOTS with Solutions for CBSE Class 9 Science

Frequently Asked Questions (FAQs) on Surface Areas and Volumes Case Study