Fractions and Decimals Class 7 Case Study Questions Maths Chapter 2

Reading Time: 8 minutes

Last Updated on August 17, 2024 by XAM CONTENT

Hello students, we are providing case study questions for class 7 maths. Case study questions are the new question format that is introduced in CBSE board. The resources for case study questions are very less. So, to help students we have created chapterwise case study questions for class 7 maths. In this article, you will find case study questions for CBSE Class 7 Maths Chapter 2 Fractions and Decimals. It is a part of Case Study Questions for CBSE Class 7 Maths Series.

Chapter	Fractions and Decimals
Type of Questions	Case Study Questions
Nature of Questions	Competency Based Questions
Board	CBSE
Class	7
Subject	Maths
Useful for	Class 7 Studying Students
Answers provided	Yes
Difficulty level	Mentioned
Important Link	Class 7 Maths Chapterwise Case Study

Case Study Questions on Fractions and Decimals

Questions

Passage 1:

Insulin is a hormone made by the pancreas in the human body. It helps to regulate blood sugar level and absorbs glucose to get energy. The number of required units of insulin varies according to the individual. In some cases, an individual body is not able to produce sufficient amount of insulin. For those individuals, supplementary units of insulin are injected into the body.

Difficulty Level: Medium

Q. 1. A doctor uses this formula to calculate the daily insulin units required for Prakhar’s body. Daily insulin requirement (units) = 0.55 x Total body mass (kilograms).
Prakhar’s body mass is 80 kg.
What is Prakhar’s daily insulin requirement?

Ans. 44 units (0.55 x 80)

Q. 2. Insulin also helps in disposing of carbohydrates in the human body. In Prakhar’s body, 14.5 insulin units dispose of 290 grams of carbohydrate. How many grams of carbohydrates are disposed of by 1 insulin unit?
(a) 200
(b) 20
(c) 2
(d) 0.2

Ans. Option (b) is correct.

Passage 2:

Some balls have been assigned a tag with decimal numbers according to the weight of the ball. The balls are to be placed on the basis of the following:

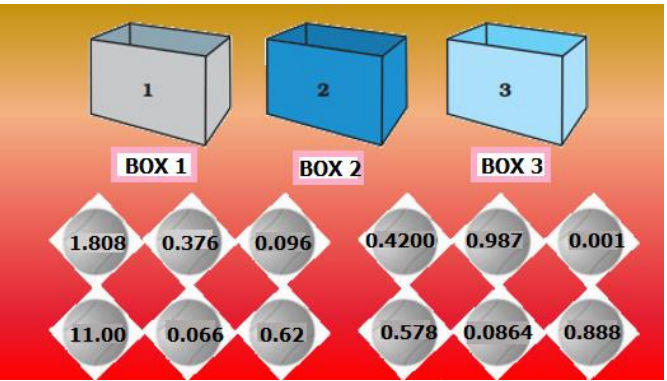
– All balls with tag number less than $\frac{1}{8}$ should be placed in box 1.
– All balls with tag number between $\frac{3}{8}$ and $\frac{5}{8}$ should be placed in box 2.
– All balls with tag number more than $\frac{7}{8}$ should be placed in box 3.

Place the balls in the appropriate boxes.
(Hint: Convert $\frac{1}{8}, \frac{3}{8}, \frac{5}{8}$ and $\frac{7}{8}$ in to decimal numbers.

(i) The number of balls placed in box 1 is
A	5	B	3	C	4	D	6
(ii) If there is a ball with a tag 0.795, then it can be placed in which of these boxes?
A	1	B	2	C	3	D	None of these
(iii) What is the total weight of balls that are placed in box 3 ?
A	14.683 Kg	B	1.993 Kg	C	0.2494 Kg	D	146.83 Kg
(iv) What will be the quotient when you divide 1.993 by 5 ?
A	39.86	B	3.986	C	0.3986	D	398.6
(v) What is the weight of 10 such boxes of type 3?
A	14.683 Kg	B	146.83 Kg	C	1468.3 Kg	D	14683 Kg

Answers 2:

(i) C
(ii) D
(iii) A
(iv) C
(v) B

Also check

• Visualizing Solid Shapes Class 7 Case Study Questions Maths Chapter 13
• Symmetry Class 7 Case Study Questions Maths Chapter 12
• Exponents and Powers Class 7 Case Study Questions Maths Chapter 11
• Algebraic Expressions Class 7 Case Study Questions Maths Chapter 10
• Perimeter and Area Class 7 Case Study Questions Maths Chapter 9
• Rational Numbers Class 7 Case Study Questions Maths Chapter 8
• Comparing Quantities Class 7 Case Study Questions Maths Chapter 7
• Triangle and its Properties Class 7 Case Study Questions Maths Chapter 6
• Lines and Angles Class 7 Case Study Questions Maths Chapter 5
• Simple Equations Class 7 Case Study Questions Maths Chapter 4
• Data Handling Class 7 Case Study Questions Maths Chapter 3
• Fractions and Decimals Class 7 Case Study Questions Maths Chapter 2
• Integers Class 7 Case Study Questions Maths Chapter 1

Topics from which case study questions may be asked

• Multiplication of Fractions
• Division of Fractions
• Multiplication of Decimals
• Division of Decimals

Numbers of the form p/q, where q ≠ 0 are called fractions, p is the numerator and q is the denominator. A fraction in which p < q is proper fraction and a fraction in which p > q is called an improper fraction.

Case study questions from the above given topic may be asked.

Fraction whose denominator is a multiple of 10 is called a decimal fraction.

Frequently Asked Questions (FAQs) on Fractions and Decimals Case Study