Numerical Problems on Convex and Concave Mirror with Answers

Download CBSE and ICSE Books in PDF Format

Reading Time: 11 minutes

Last Updated on August 31, 2024 by XAM CONTENT

Hello students, here you will find numerical problems on convex and concave mirror with answers. These numericals on convex and concave mirror are very useful for students studying in CBSE Class 10 or for anyone who wants to practice some problems on these topics.

Before we start solving the numerical problems, let’s see some important points and formulae. This will help you to solve the problems efficiently.

Customised Study Materials for Teachers, Schools and Coaching Institute

Table of Contents

Important Concept and Formulae

(1) The distance of the principal focus from the pole of the mirror is called the focal length of the mirror. It is denoted by f. If R is radius of curvature of the mirror of focal length, we can write

R = 2 f

(2) Mirror formula is the relation between the focal length (f)of the mirror, the distance u of the object from the pole of the mirror and the distance v of the image from the pole.

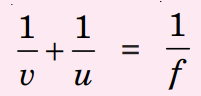
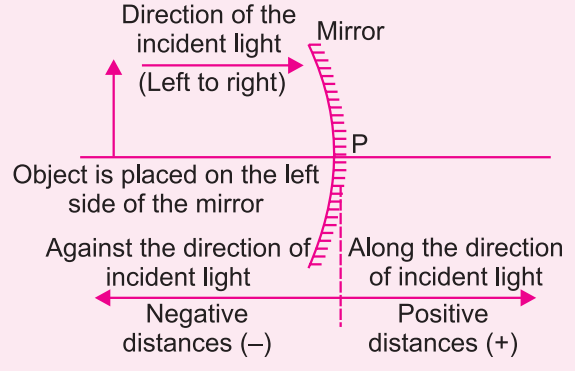
(3) New cartesian sign convention
In case of spherical mirrors, the following sign convention is used for measuring various distances:
(i) All distances are measured from the pole of the mirror.
(ii) All distances measured in the direction of the incident ray are taken as positive.
(iii) All distances measured in the direction opposite to that of the incident ray are taken as negative.
(iv) The distances measured above the principal axis are taken as positive.
(v) The distance measured below the principal axis are taken as negative

Now you can start solving the numerical problems given below. Answers are given along with questions so that you can check quickly after solving a particular problem.

File size

Convex and Concave Mirror Numerical Problems with Answers

Q.1. An object is placed at a distance of 10 cm from the pole of a concave mirror. Its image is formed at 6 cm from its pole. Calculate the focal length of the mirror.

Ans. f = –3.75 cm.

Q.2. An object is placed at a distance of 6 cm from a convex mirror of focal length 12 cm.

Ans. v = 4 cm, Nature: Virtual, erect and small in size.

Q.3. A concave mirror produces a real image of height 2 cm of an object from 0.5 cm placed 10 cm away from the mirror. Find the position of the image and focal length of the mirror?

Ans. f = –8 cm and v = –40 cm

Q.4. A dentist uses a small concave mirror of focal length 3.0 cm and holds it at a distance of 2 cm from the tooth. What is the magnification of the image?

Ans. m = 3, the image is three times the size of the object

Q.5. How far should an object be held from a concave mirror of focal length 40 cm so as to get an image magnified three times?

Ans. If the object is held at a distance of 53.33 cm, a real image thrice in size is obtained. Also, a virtual image of thrice the size is obtained if the distance of the object is 26.67 cm.

Q.6. A concave mirror of focal length 10 cm is kept in front of an object at a distance of 50 cm from it. If the object is 1.0 cm high, what will be the size of the image?

Ans. The size of the image is 0.25 cm.

Q.7. How far from a lamp must a concave mirror of focal length 3.0 m be placed in order to throw its image on the screen 8.0 m from the lamp?

Ans. u = -4.8 cm

Q.8. An object is located 20 cm in front of a concave mirror of radius of curvature 60 cm. Find the position and nature of the image formed.

Ans. v = 60 cm, virtual, erect and enlarged.
m = 3, Image is 3 times the size of the object.

Q.9. An object 5 cm in height is placed in front of a concave mirror on the principal axis at a distance of 10 cm from the mirror. If the focal length of the mirror is 20 cm, find the nature of the image formed.

Ans. Virtual, erect and magnified image is formed behind the mirror.

Q.10. An object 10 cm tall is placed on the principal axis of a convex mirror of focal length 30 cm at a distance of 20 cm from the pole. Find the nature, size, position and magnification of the image formed on the principal axis (by graphical construction).

Ans. Virtual, erect and diminished image (m = 0.6) is formed behind the mirror.

Q.11. Find the size, nature and position of image formed when an object of size 1 cm is placed at a distance of 15 cm from a concave mirror of focal length 10 cm.

Ans. Position of image is 30 cm to the left side of mirror or 30 cm in front of the mirror.

Nature of image: As the image is formed in front of the concave mirror, it is ‘real and inverted’.

Size of image: The image is 2 cm long and is formed below the principal axis.

Q.12. An object 2 cm high is kept at a distance of 16 cm from a concave mirror which produces a real image 3 cm high.
(i) What is the focal length of the mirror?
(ii) Find the position of the image.

Ans. The image is formed 24 cm in front of the mirror. The focal length of the concave mirror is –9.6 cm.

Q.13. An object 5 cm high is placed at a distance of 10 cm from a convex mirror of radius of curvature 30 cm. Find the nature, position and size of the image.

Ans. The image is formed 6 cm behind the convex mirror. The image being behind the mirror, it is virtual and erect. The size of the image is 3 cm.

Q.14. What is the position of an image when an object is placed at a distance of 20 cm from a concave mirror of focal length of 20 cm?

Ans. The image is formed at infinity.

Q.15. A concave mirror of focal length 30 cm is placed at a distance of 90 cm from the wall. How far from the wall should an object be placed so as to get its real image on the wall?

Ans. The object is at a distance of 45 cm from the mirror (90 cm – 45 cm) from the wall.

Q.16. An object placed 50 cm from a lens produces a virtual image at a distance of 10 cm in front of the lens. What is the focal length of the lens? Is it converging or diverging lens?

Ans.

Given:

$$
\begin{aligned}
& u=-50 \mathrm{~cm} \\
& v=-10 \mathrm{~cm} \\
& f=?
\end{aligned}
$$

According to the lens formula,

$$
\frac{1}{v}-\frac{1}{u}=\frac{1}{f}
$$

Substituting the values and solving we get,

$$
\text { or } \begin{aligned}
-\frac{1}{10}-( & \left.-\frac{1}{50}\right)=\frac{1}{f} \\
& -\frac{1}{f}=\frac{1}{50}-\frac{1}{10} \\
& =\frac{1-5}{50}=\frac{-4}{50}=\frac{-2}{25}
\end{aligned}
$$

or $\quad f=-12.5 \mathrm{~cm}$
Since focal length is negative, the lens must be a diverging lens or concave lens.

Q.17. An object is placed at a distance of 4 cm from a concave lens of focal length 12 cm. Find the position and nature of the image formed.

Ans.

Using the lens formula,

$$
\frac{1}{v}-\frac{1}{u}=\frac{1}{f}
$$

Putting the given values and solving we get,

$$
\begin{aligned}
& \frac{1}{v}=\frac{1}{u}+\frac{1}{f} \\
& =-\frac{1}{4}-\frac{1}{12} \\
& =\frac{-3-1}{12}=\frac{-4}{12} \\
& \text { or } \\
& v=-3 \mathrm{~cm} \\
& \text { Magnification ( } m \text { ) } \\
& =\frac{v}{u} \\
& =\frac{-3}{-4} \\
& =0.75
\end{aligned}
$$
The image formed by the lens is at a distance of 3 cm from the lens on its left side. The image formed is virtual, erect and smaller in size than the object.

File size

Helpful Links for CBSE Class 10 Science Preparation

Also check

Quiz

Topic	Light – Reflection and Refraction of Light
Useful for	CBSE Class 10 Students
Type of Questions	MCQs
No. of Questions	40
Solutions	Instant Solutions after Completion of Quiz
Quiz Link	Click here to Take Test
Price	Free

How to take quiz or test using the given link

It’s quite simple!

Step 1: Click on the given link. You will see the below screen.

Step 2: Fill in the necessary details. There is no need to register. Just fill your email and name and click on the button “Take Assessment”. The below screen will appear.